high-resolution terminology - matching measurements at high-resolution

Flux control efficiency

Description

Flux control efficiencies express the control of respiration by a metabolic control variable, X, as a fractional change of flux from Y_X to Z_X, normalized for Z_X. Z_X is the reference state with high (stimulated or un-inhibited) flux; Y_X is the background state at low flux, upon which X acts.

j_Z-Y = (Z_X-Y_X)/Z_X = 1-Y_X/Z_X

Complementary to the concept of flux control ratios and analogous to elasticities of metabolic control analysis, the flux control efficiency of X upon background Y_X is expressed as the change of flux from Y_X to Z_X normalized for the reference state Z_X. » MiPNet article

Abbreviation: j_Z-Y

Reference: Gnaiger 2020 BEC MitoPathways

Flux control efficiency: normalization of mitochondrial respiration

Gnaiger E (2020) Flux control efficiency: normalization of mitochondrial respiration. Mitochondr Physiol Network 2016-03-20; updated 2020-11-10.

» Gnaiger 2020 BEC MitoPathways

Oroboros (2020) MiPNet

Abstract: The flux control efficiency, j_Z-Y, and flux control ratio, FCR, are internal normalizations, expressing respiratory flux in a given state relative to respiratory flux in a reference state. Whereas FCRs express various respiratory states relative to a common refrence state, j_Z-Y express the control of respiration in a single step caused by a specific metabolic control variable X. The concept of the flux control efficiency presents a generalized framework for assessing the effect of an experimental variable on flux and defines specific expressions, such as the biochemical coupling efficiency.

• O2k-Network Lab: AT Innsbruck Gnaiger E

Metabolic control variable and respiratory state

A metabolic control variable X is either added (stimulation, activation) or removed (reversal of inhibition) to yield a high flux Z in the reference state, compared to flux Y in the background state. X denotes the metabolic control variable; Y and Z are the respiratory states, whereas Y and Z denote the corresponding respiratory fluxes. j_Z-Y in step analysis relates to the change of flux caused by the variable X. The FCR in state analysis compares fluxes in a variety of respiratory states which may be separated by single or multiple variables, i.e. separated by several coupling and pathway control states.

If inhibitors are experimentally added rather than removed (-X); then Y_X is the background rate in the presence of the inhibitor.

X: Metabolic control variable acting on Y_X in the background state, to yield rate Z_X in the reference state. X stimulates or un-inhibits Y_X from low flux to Z_X at high flux.
Y_X: The rate in the background state Y is the non-activated or inhibited respiratory rate (low) in relation to the high rate Z_X in the reference state Z. A metabolic control variable, X, acts on Y_X (substrate, activator) or is removed from Y (inhibitor) to yield Z_X. The X-specific (in contrast to general) flux control ratio is Y/Z.
Z_X: The rate in the reference state Z, stimulated or un-inhibited by a metabolic control variable, X, with high rate in relation to rate Y_X in the background state Y.

Pathway control efficiency

Pathway control efficiencies express the relative change of oxygen flux in response to a transition of (1) CHNO-fuel substrates or (2) inhibitors of enzyme steps in the pathway, in a defined coupling state.

» NS-N pathway control efficiency

Coupling-control efficiency

Coupling-control efficiencies are determined in an ET-pathway competent state. The terms coupling efficiency and coupling-control efficiency are used synonymously.

mt-Preparations

In mitochondrial preparations, there are three well-defined coupling states of respiration, L, P, E (LEAK respiration, OXPHOS, Electron transfer pathway).

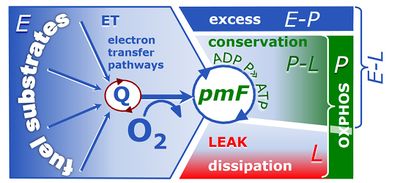
1. If the metabolic control variable, X, is an uncoupler, the reference rate Z_X is E. Then two background states Y, of coupling control are possible: The uncoupler may act on L or P in mt-preparations. The corresponding flux control efficiencies are:

E-L coupling efficiency, j_E-L = (E-L)/E = 1-L/E.
E-P control efficiency, j_E-P = (E-P)/E = 1-P/E.

2. If the metabolic control variable is stimulation by ADP, D, or release of an inhibitor of phosphorylation of ADP to ATP (DT-phosphorylation; e.g. -Omy), the reference rate Z is P at saturating concentrations of ADP. The background rate Y is L, and the corresponding flux control efficiency is:

P-L control efficiency, j_P-L = (P-L)/P = 1-L/P, related to the RCR.

3. If the background rate Y is L, the metablic control variable from L to P is ADP-saturated ATP turnover or release of an inhibitor of phosphorylation of ADP to ATP, and the reference rate Z is E, the coupling control ratio is expressed as the net P/E control ratio (compare 1 and 2):

(P-L)/E

Living cells

L(Omy) and E can be induced in living cells, but rate P cannot. However, the ROUTINE rate of respiration R can be measured in living cells.

1. If the metabolic control variable X is an uncoupler, the reference rate Z is E. Then two background states, Y of coupling control are possible: The uncoupler may act on the LEAK respiration or ROUTINE state in living cells. The corresponding coupling control efficiencies are:

E-L coupling efficiency, j_E-L = (E-L)/E = 1-L/E.
E-R control efficiency, j_E-R = (E-R)/E = 1-R/E.

2. If the metabolic control variable is stimulation by ATP turnover or release of an inhibitor of phosphorylation of ADP to ATP (DT-phosphorylation; e.g. -Omy), the reference rate Z is R in living cells at physiologically controlled steady states of [ADP] and ATP-turnover. The background rate Y is L, and the corresponding flux control efficiency is:

R-L control efficiency, j_R-L = (R-L)/R = 1-L/R.

3. If the background rate Y is L, the metablic control variable from L to R is cell-controlled ATP turnover or release of an inhibitor of phosphorylation of ADP to ATP, and the reference rate Z is E, the coupling control ratio is expressed as the net R/E control ratio (compare 1 and 2):

(R-L)/E

References

Keywords

Expand Bioblast links to Flux control efficiency

```
The Blue Book 2020
```
OXPHOS-, ROUTINE-, ET-, and LEAK states; respiratory capacities (P, R, E, L) corrected for residual oxygen consumption Rox.
4-compartmental OXPHOS model. (1) ET capacity E of the noncoupled electron transfer system ETS. OXPHOS capacity P is partitioned into (2) the dissipative LEAK component L, and (3) ADP-stimulated P-L net OXPHOS capacity. (4) If P-L is kinetically limited by a low capacity of the phosphorylation system to utilize the protonmotive force pmF, then the apparent E-P excess capacity is available to drive coupled processes other than phosphorylation P» (ADP to ATP) without competing with P».
(P-L)/P is the OXPHOS P-L control efficiency. The biochemical coupling efficiency is independent of kinetic control by the phosphorylation system when expressed as the E-L coupling efficiency, (E-L)/E. (E-P)/E is the kinetic E-P control efficiency.

Bioblast links: Coupling control - >>>>>>> - Click on [Expand] or [Collapse] - >>>>>>>

1. Mitochondrial and cellular respiratory rates in coupling-control states

» Baseline state

Respiratory rate	Defining relations
OXPHOS capacity	P = P´-Rox	mt-preparations
ROUTINE respiration	R = R´-Rox	living cells
ET capacity	E = E´-Rox	» Level flow
		» Noncoupled respiration - Uncoupler
LEAK respiration	L = L´-Rox	» Static head
		» LEAK state with ATP
		» LEAK state with oligomycin
		» LEAK state without adenylates
Residual oxygen consumption Rox	L = L´-Rox

Chance and Williams nomenclature: respiratory states

» State 1 —» State 2 —» State 3 —» State 4 —» State 5

2. Flux control ratios related to coupling in mt-preparations and living cells

» Flux control ratio

» Coupling-control ratio

» Coupling-control protocol

FCR	Definition
L/P coupling-control ratio	L/P	» Respiratory acceptor control ratio, RCR = P/L
L/R coupling-control ratio	L/R
L/E coupling-control ratio	L/E	» Uncoupling-control ratio, UCR = E/L (ambiguous)
P/E control ratio	P/E
R/E control ratio	R/E	» Uncoupling-control ratio, UCR = E/L
net P/E control ratio	(P-L)/E
net R/E control ratio	(R-L)/E

3. Net, excess, and reserve capacities of respiration

Respiratory net rate	Definition	Icon
P-L net OXPHOS capacity	P-L
R-L net ROUTINE capacity	R-L
E-L net ET capacity	E-L
E-P excess capacity	E-P
E-R reserve capacity	E-R

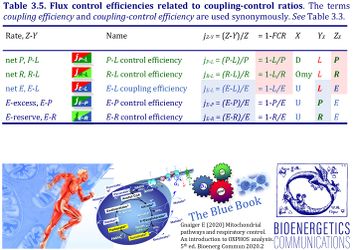
4. Flux control efficiencies related to coupling-control ratios

» Flux control efficiency j_Z-Y

» Background state

» Reference state

» Metabolic control variable

Coupling-control efficiency		Definition		Canonical term
P-L control efficiency	j_P-L	= (P-L)/P	= 1-L/P	P-L OXPHOS-flux control efficiency
R-L control efficiency	j_R-L	= (R-L)/R	= 1-L/R	R-L ROUTINE-flux control efficiency
E-L coupling efficiency	j_E-L	= (E-L)/E	= 1-L/E	E-L ET-coupling efficiency » Biochemical coupling efficiency
E-P control efficiency	j_E-P	= (E-P)/E	= 1-P/E	E-P ET-excess flux control efficiency
E-R control efficiency	j_E-R	= (E-R)/E	= 1-R/E	E-R ET-reserve flux control efficiency